Hey Ray: Pi Day probability
PITTSBURGH (KDKA) - As we have shown you in the past, with pizza, Pi is the relationship between the diameter of a circle and its circumference.
No matter how big the circle is, the circumference is 3.14 times the diameter.
That is why we celebrate Pi Day on March 14th...you know 3-1-4.
For this Pi Day, I'm going to show you the idea of Pi is not necessarily only in circles you can see.
In class, we spend our time with circles to demonstrate Pi, but we are going to use Pi to predict how toothpicks land when they are dropped.
This will show us that Pi is important to statistics and probability. Probability is something very important to forecasting!
This is an exercise called Buffon's Needle. It is named after Georges Buffon, the scientist, and mathematician who came up with it.
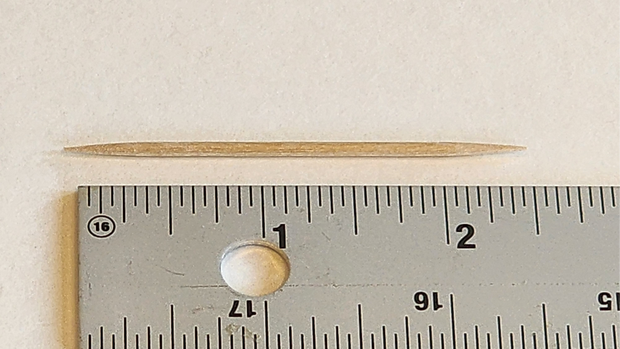
Obviously, we're using toothpicks instead of needles. Match sticks work well, too, if you're doing this at home.
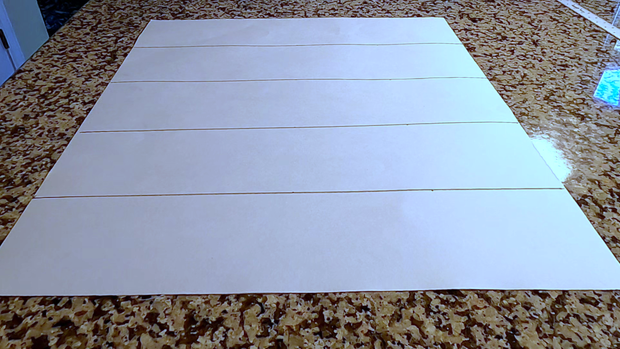
What we are going to do is drop toothpicks onto a poster board that has lines on it. Those lines are spaced apart a measurement that is twice as big as our toothpicks. So, since our toothpicks are two and a half inches long, we will want the lines to be spaced out about five inches.
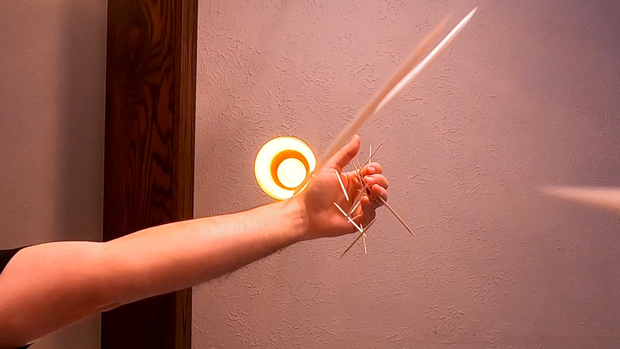
Now, we just start dropping toothpicks onto the paper.
You will want to drop enough toothpicks to get a good sample.
At least 100 toothpicks should be used.
We are going to drop 225 onto our target. After you drop your sample, just start counting how many toothpicks touch or cross a line on the paper. If a toothpick falls off the paper, just pick it up and drop it again from about the same spot you dropped the others.
From there, you just take the total number of toothpicks and divide it by the number of toothpicks that crossed a line. We had 225 total toothpicks and 72 that crossed or touched a line.
When we do the math....225 toothpicks divided by the 72 that crossed the line, we come up with 3.125 which is very close to Pi which is 3.14. The more toothpicks or match sticks you use, the closer to the actual Pi your final number will become.
You can make the prediction that no matter how many times you perform this experiment with a good sample, you will keep getting a very close estimate of Pi!
It makes you wonder about the other places Pi is hidden.